

Relate the importance of angular diameter for measuring diametersof objects in solar system.Describe how the distance between the moon and the earth affectsthe angular distance of the moon and the sun.Explain why the angular distance between the moon and the sun arethe same during a total lunar eclipse.
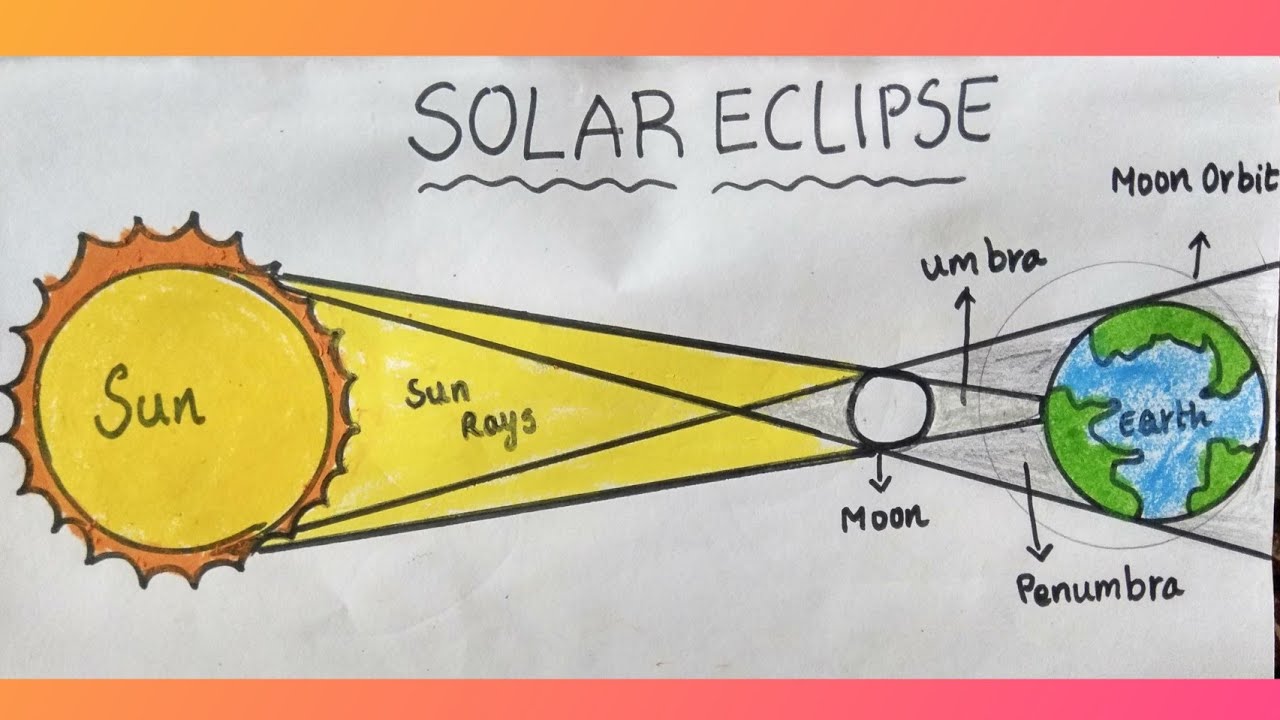
Use their knowledge of proportion and right triangles tocalculate the furthest distance that the moon can be from the earth (given afixed distance from the earth to the sun) in order to observe a total solareclipse.Describe how the orbit of the moon has an impact upon the phasesof the moon as witnessed from Earth.The students will then explore how the angular distanceof the moon and sun from the earth during a total solar eclipse are impacted bythe earth-moon and earth-sun distances. After reviewing the orbit of themoon around the sun, as demonstrated through a discussion of moon phases, thestudents will explore how the relationship between the earth-moon distance andthe earth-sun distance plays a critical role in determining the possibility ofa total solar eclipse. This lesson provides studentswith the opportunity to apply their knowledge of proportions and angles to thestudy of real phenomena – solar eclipses. (f)(3) In avariety of ways, the student develops, applies, and justifies trianglesimilarity relationships, such as right triangle ratios, trigonometric ratios,and Pythagorean triples. (f)(2)The student uses ratios to solve problems involving similar figures. (f)(1) Thestudent uses similarity properties and transformations to explore and justifyconjectures about geometric figures.

Students perceive theconnections between algebra and geometry and use the tools of one to help solveproblems in the other. Equations and functionsĪre algebraictools that can be used to represent geometric curves and figures similarly,geometric figures can illustrate algebraic relationships. (a)(4)Relationship between algebra and geometry. They may require a little more prompting when usingright triangles and proportions to do the activity, but similar triangles,basic ratio and proportion, and the Pythagorean theorem are all covered in 8 thgrade mathematics. §111.33.Algebra II Do you realize you will be teachingAlgebra I students and not Alg II students? After looking over the lesson, Idon’t think there is anything in it that Algebra I kids cannot do (unless theyhave to use trig ratios).
#SIMPLE DIAGRAMS FOR LUNAR AND SOLAR ECLIPSE SERIES#
Sources ofthe Lesson: Our SolarSystem: Hands-on Science Series (Kwitter, Souza, 1999), Project Earth Science: Astronomy (Smith, 2001). Authors: Julie Andersen, Shelly Rogers, and SadiaWaheedĭate ofLesson: MaMarch 30, 2005


 0 kommentar(er)
0 kommentar(er)
